Torque

 

Power is the rate at which work is done, that is the rate at which energy is transferred by force. The rotational force that is generated by the engine (by which the energy is transferred) is called torque.

RPM is the short for "rotation per minute". This is the speed at which the engine is rotating the flywheel.

Internal combustion (IC) engines do not produce same power at all engine speeds, hence there would be an engine speed (RPM) at which the engine would be generating max power. Same is the case with torque.
As a characteristic of IC engine the max torque is normally produced at a lesser engine speed than when the max power is.

In much simpler terms.
Power is the amount of energy that the engine can transfer with respect to time... (That's where you get your pickup from)
Torque is the load carrying capacity.
So it depends on your need. The more acceleration you want, the more power you need... whereas, in case u need to carry load around you need more torque.
The rpm indicates at what rpm the engine can deliver the max power/torque.
Petrol cars are good in delivering their power, whereas diesel engines in delivering their torque.
For accelerating you need both power and torque. In very simple terms, torque is the rate at which an engine can produce power. Remember, acceleration is the rate at which speed changes, so to maintain a speed you need power to overcome the resistance (road friction, air friction and friction in the moving parts of the car engine and transmission as well). Normally, the RPM between the max torque and max power is the power band of the car, lower end of this band is where you would be cruising, and higher end is where you are racing.

And now let's get bit more technical.

Rotational Torque (T, Nm) is the product of Rotational Mass (J, Inertia) x (Angular Acceleration, Change in Velocity over the Change in Time, dw/dt). (T = J x (dw/dt) or (F x Radius)), in the same way as Linear Force is the product of Mass (Kg) x (Acceleration m/s^2). The F x Radius will become more important later.

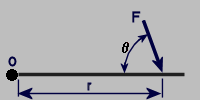
Torque is a measure of how much force acting on an object causes that object to rotate. The object rotates around an axis, which we will call the pivot point, and will label 'O'. We will call the force 'F'. The distance from the pivot point to the point where the force acts is called the moment arm, and is denoted by 'r'. Note that this distance, 'r', is also a vector, and points from the axis of rotation to the point where the force acts. (Refer to Figure 1 for a picture representation of these definitions.)

You generate a torque any time you apply a force using a wrench. Tightening the lug nuts on your wheels is a good example. When you use a wrench, you apply a force to the handle. This force creates a torque on the lug nut, which tends to turn the lug nut.
The SI unit for torque is the Newton meter (Nm). In Imperial and U.S. customary units, it is measured in foot pounds (ft·lbf) (also known as 'pound feet') and for smaller measurement of torque: inch pounds (in·lbf) or even inch ounces (in·ozf).

Torque is defined as:

r X F =r F sin

 

Notice that the torque units contain a distance and a force. To calculate the torque in Imperial units, you just multiply the force by the distance from the center. In the case of the lug nuts, if the wrench is a foot long, and you put 200 pounds of force on it, you are generating 200 pound-feet of torque. If you use a 2-foot wrench, you only need to put 100 pounds of force on it to generate the same torque.
In SI units, a force of three Newton's applied two meters from the lug nuts, for example, exerts the same torque as one Newton applied six meters from the lug nuts. This assumes, in both cases of course, the force is in a direction at right angles to the straight lever.

A car engine creates torque and uses it to spin the crankshaft. This torque is created exactly the same way: A force is applied at a distance. Let's take a close look at some of the engine parts:

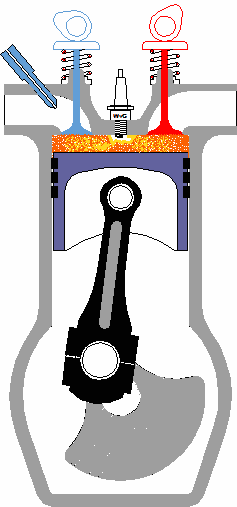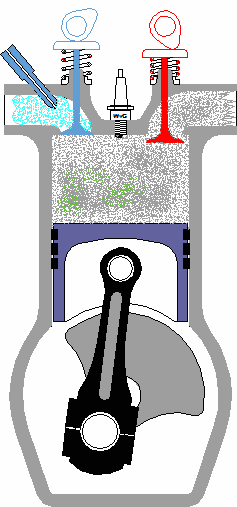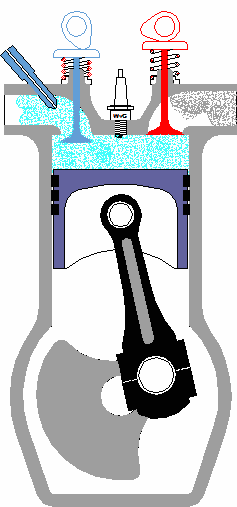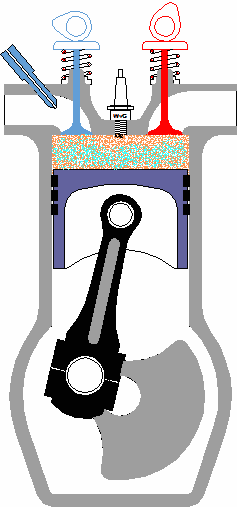
The combustion of gas in the cylinder creates pressure against the piston. That pressure creates a force on the piston head, which pushes it down. The force is transmitted from the piston to the connecting rod, and from the connecting rod into the crankshaft. Notice that the point where the connecting rod attaches to the crankshaft is some distance from the center of the shaft. The horizontal distance changes as the crankshaft spins, so the torque also changes, since torque equals force multiplied by distance.
You might be wondering why only the horizontal distance is important in determining the torque in this engine. You can see that when the piston is at the top or bottom of its stroke, the connecting rod points straight down or up at the center of the crankshaft. No torque is generated in this position, because only the force that acts on the lever in a direction perpendicular to the lever generates a torque.

 

 

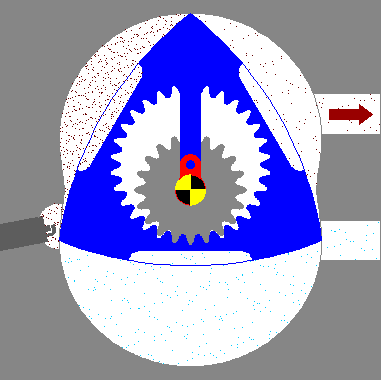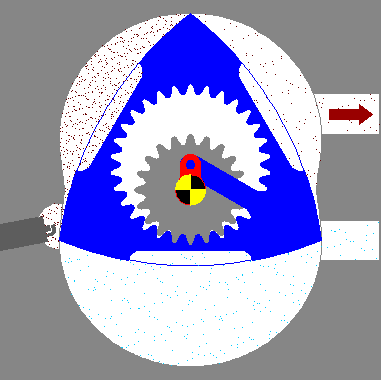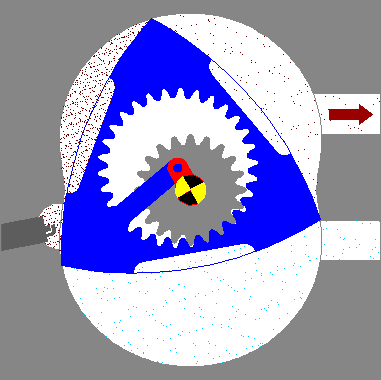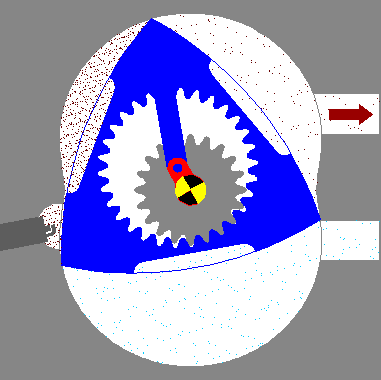
In the same way you can describe work of Roto Wankel engine. In the above picture you can see blue "connecting rod" connected to center shaft . As angle is changing, torque is changing, but is rarely happening that "blue connecting rod" points straight at the center of the crankshaft. Actually, every third rotation. Otto engine does this twice every rotation. And as I explained before, that is a moment when torque is 0. That's why Wankel engine has better torque and power than Otto engine.

A torque in physics is a pseudo vector that measures the tendency of a force to rotate an object about some axis (or fulcrum or pivot). Just as a force is a push or a pull, a torque can be thought of as a twist. The symbol for torque is , the Greek letter tau.

Torque is also called moment or moment of force. This should not be confused with the various other definitions of "moment" in physics. In the context of mechanical engineering, the terms "moment" and "torque" are not necessarily interchangeable; rather, one or the other are preferred in a specific context. For example, "torque" is usually used to describe a rotational force down a shaft, for example a using a wrench, whereas "moment" is more often used to describe a bending force on a beam.

The magnitude of torque depends on three quantities: First, the force applied; second, the length of the lever arm connecting the axis to the point of force application; and third, the angle between the two. Again, in symbols:

Where

	is torque vector
	is lever arm vector (vector from the axis to point of force application and the r is length of the moment arm
	is the force vector, and F is the magnitude of force
X	denotes cross product
	is the angle between the force vector and moment arm vector
	magnitude of torque

The length of the lever arm is particularly important; choosing this length appropriately lies behind the operation of levers, pulleys, gears, and most other simple machines involving a mechanical advantage

The direction of the torque can be determined by using the right hand grip rule: curl the fingers of your right hand to indicate the direction of rotation, and stick your thumb out so it is aligned with the axis of rotation. Your thumb points in the direction of the torque vector.

Torque is part of the basic specification of an engine: the power output of an engine is expressed as its torque multiplied by its rotational speed of the axis. Internal-combustion engines produce useful torque only over a limited range of rotational speeds (typically from around 2,000-5,000 rpm for a small car). The varying torque output over that range can be measured with a dynamometer, and shown as a torque curve. The peak of that torque curve occurs somewhat below the overall power peak. The torque peak cannot, by definition, appear at higher rpm than the power peak.

Understanding the relationship between torque, power and engine speed is vital in automotive engineering, concerned as it is with transmitting power from the engine through the drive train to the wheels. Power is typically a function of torque and engine speed. The gearing of the drive train must be chosen appropriately to make the most of the motor's torque characteristics.

Typically a turbo engine torque curve is a steep rise and then flat to near max revs. A typical atmosferic engine is very different, with very low torque at low RPM and then a rapid rise to peak torque RPM and then again rapid drop. The area under the Turbo torque curve is far far larger with a turbo engine. And if you double the torque you double the acceleration, take the gear box out of it. Torque has a role in improving acceleration for exactly the reasons I have outlined about the torque curve: more gears allows the designer to keep the engine more closely within its peak torque production rpm. Atmosferic engine needs revs to produce power.

If a force is allowed to act through a distance, it is doing mechanical work. Similarly, if torque is allowed to act through a rotational distance, it is doing work. Power is the work per unit time. However, time and rotational distance are related by the angular speed where each revolution results in the circumference of the circle being travelled by the force that is generating the torque.
The power injected by the applied torque may be calculated as:

Power = Torque x Angular Speed

As you can see torque and power are directly related. Power is simply the application of X amount of torque for a Y amount of time. Consistent units must be used. For metric SI units power is watts, torque is newton meters and angular speed is radians per second (not rpm and not revolutions per second).
On the right hand side, this is a scalar product of two vectors, giving a scalar on the left hand side of the equation. Mathematically, the equation may be rearranged to compute torque for a given power output. Note that the power injected by the torque depends only on the instantaneous angular speed - not on whether the angular speed increases, decreases, or remains constant while the torque is being applied (this is equivalent to the linear case where the power injected by a force depends only on the instantaneous speed - not on the resulting acceleration, if any).

For different units of power, torque or angular speed, a conversion factor must be inserted into equation. Also, if rotational speed (revolution per time) is used in place of angular speed (radians per time), a conversation factor of 2Pi must be added because there are 2Pi radians in one revolution:

Power = torque X 2π X rotational speed

where rotational speed is in revolutions per unit time.

Now you can calculate power of your car at given RPM-s. And now you can understand why Formula 1 is so thirsty for engine speed. The "easiest" way to increase the power is to increase the engine rotational speed.

Aa, BTW, Formula 1!

In Formula 1 engineers tend to map torque level of the engine for separate circuits, for different parts of the circuit and different conditions on the racing track. Engine maps are one of the dark arts of F1. There is no use of high torque value on the wet track. An engine torque map represents the torque delivered by the engine as a function of engine speed and engine throttle position. In the ECU, the engine torque map is used to position the engine throttles to match the drivers’ torque demand. The driver torque map represents the torque requested by the driver as a function of engine speed and accelerator pedal position. FIA introduced regulations that govern how you may control engine torque, and is covered by Articles 5.5 and 5.6 of the technical regulations.
Except for some specific exceptions, the engine torque must be controlled by the driver. These exceptions include: downshifts, pit lane speed limiter, anti-stall function and the end of straight limiter strategy. Note that not all exceptions are listed here. There are many more.
The driver may only control the torque by means of a single accelerator pedal.
At zero per cent pedal (off throttle), the torque demand must be less than or equal to zero; at one hundred per cent pedal (full throttle), the torque demand must match or exceed the maximum torque output of the engine in its current state (Article 5.5.3).
There are limits on the shape of the torque demand as a function of pedal position and engine speed (to prevent engine characteristics that could be used as driver aids).
Respecting these restrictions, the torque demand is shaped against throttle position and engine speed to deliver the desired response for the driver and car.
Driver pedal maps can be changed from circuit to circuit as a function of the circuit characteristics. For example, drivers might want more precision during initial pedal application at Monaco. Similarly, some drivers insist on a wet weather pedal map.
The engine torque maps are also adjusted to take account of the engine’s power output according to the ambient conditions. The engines will all produce more torque on a cold day at Silverstone than at Interlagos (low air pressure) or Malaysia (high specific humidity). This ensures that the drivers feel the same engine response at part load, regardless of weather.
Torque maps may also change as a consequence of changes to exhausts or air inlet (if teams introduce a new exhaust design or new air box).

 

Steam engines and electric motors (especially DC electric motors - Direct Current) tend to produce maximum torque close to zero rpm, with the torque diminishing as rotational speed rises.

To know more, please read my articles about torque map.

 

Back to the top of the page